An Analytic Hierarchy Process for a General Ranking of Friction Stir Welding Parameters Optimized Through the Design of Experimental Arrays

 

Mohamed Mohamed Abd Elnabi1*, Abdelrahman Abdelaal1

 

1Department of Mechanical Design and Production, Faculty of Engineering, Cairo University, Giza, Egypt

 

*Correspondence to: Mohamed Mohamed Abd Elnabi, PhD student, Department of Mechanical Design and Production, Faculty of Engineering, Cairo University, 1 Gamaa Street, Giza 12613, Egypt; Email: mohamed.abd.M@eng-st.cu.edu.eg

 

DOI: 10.53964/jmim.2022005

 

Abstract

Objective: This study aims to rank friction stir welding parameters using the analytic hierarchy process (AHP).

 

Methods: The proposed approach includes two phases to achieve a general ranking of the parameters through the separate estimation of their weights on each metal type and aggregation. The criteria used in the AHP for ranking are the contribution percentages and the degrees of the parameters obtained from the analysis of variance of experimental studies. The data were obtained from literature that used the design of experimental arrays to optimize these parameters on multiple properties and many similar and dissimilar metals. Fourteen parameters were assigned in the present analysis, namely rotational speed (RS), traverse speed (TS), axial force (AF), pin profile (PP), tilt angle (TA), the ratio between shoulder diameter and pin diameter (D/d ratio), shoulder diameter (SD), pin diameter (Pd), plunge depth (PD), pin length (PL), tool offset (TO), shoulder concavity (SC), number of passes (NOP) and base metal side (BMS).

 

Results: The parameters were classified into highly significant, moderately significant, and insignificant based on their rankings. The RS, TS, TA, and PP were the highest-ranking parameters with a total contribution of 83.4% to outputs. The insignificant parameters included the D/d ratio, BMS, SC, AF, and Pd, with a low total contribution of 14%. The moderately significant parameters were the TO, NOP, SD, PD, and PL.

 

Conclusion: The above-mentioned significant parameters could be controlled to maximize the response. The failure to achieve the target specified in the standard by controlling only highly significant parameters signifies a need for further modification of the FSW process. Therefore, parameters of moderate significance are potential parameters that need to be controlled to achieve the target. To limit the selection between these parameters, reference should be made to the type of metal used and then the parameters with the greatest impact on the metal are selected, i.e. controlling PL for aluminum, NOP, and SD for magnesium, and TO for aluminum-to-steel and aluminum-to-copper.

 

Keywords: friction stir welding, optimization, analytic hierarchy process, ultimate tensile strength, orthogonal arrays, analysis of variance

 

1 INTRODUCTION

Friction stir welding (FSW) is a modern consolidating technique in which a rotating profiled tool consisting of a shoulder and a pin is used for heat generation. The tool is plunged into clamped materials at an extremely small thinning of 0.1-0.2mm. The friction and axial force (AF) play a crucial role in the heat generation, and a solid-state joint is achieved through traversing the tool in the direction of the required weld path. The shoulder yields the most frictional heat required to plasticize the materials, and the pin completes the heat generation in the base region and stirs the plasticized materials from side to side to perform consolidation. Immense challenges exist in joining soft metals that are susceptible to fusion problems, such as aluminum alloys, and in joining dissimilar metals due to their plain difference in physical properties and melting temperatures. FSW exhibits great success in various industries, such as automotive, marine, and aerospace, due to its distinct features and benefits over fusion processes. Solid-state techniques such as FSW are available to overcome various flaws related to fusion processes, such as hot cracking, distortion, and porosity. FSW performs under the melting temperature, about 10-20%, of the materials[1] and has been vastly utilized for welding similar or dissimilar metals for aluminum and its alloys[2-45], titanium alloys[46], magnesium alloys[47-52], steel and its alloys[53,54], copper and its alloys (i.e., brass)[55,56], dissimilar joints of aluminum to copper or brass[57-65], aluminum to steel[66-72], aluminum to magnesium[73], and steel to copper[74]. These metals are regarded as hard to weld by traditional fusion processes.

 

The FSW parameters contribute to the formation of the weld zone, which influences the microstructures and mechanical properties of the joints. The optimization of FSW parameters secures the optimum properties of the joints. Optimization of the parameters has been conducted using various methods, such as the design of experiments (DOE)[4], response surface methodology (RSM)[2], fuzzy-logic technique[75], and artificial neural network[76]. The DOE technique has been used to optimize the FSW parameters as it minimizes time and costs through a few trials. Orthogonal arrays, characterized by a small fraction of full factorial, are the main element of the DOE techniques. Analysis of variance (ANOVA) on the DOE arrays allows for the estimation of the significance and percentage of contribution of parameters on required effects[5].

 

In the present study, the FSW parameters were ranked and weighed using the Analytic hierarchy process (AHP). Fourteen parameters of FSW were assigned , namely rotational speed, rpm (RS); traverse speed, mm/min (TS); AF, KN; pin profile (PP), tilt angle (TA); the ratio between shoulder and pin diameters (D/d ratio); shoulder diameter, mm (SD), pin diameter, mm (Pd); plunge depth, mm (PD); pin length, mm (PL); tool offset, mm (TO); and base metal side (BMS), based on tool rotation direction, no of passes (NOP), and shoulder concavity (SC). This study firstly ranked the parameters separately of each metal joining group based on the types of metals. Eight sets were selected, namely aluminum and its alloys[2-45], magnesium alloys[47-52], steel and its alloys[53,54], copper and its alloys (i.e., brass)[55,56], dissimilar joints of aluminum to copper and their alloys[57-65], aluminum alloys to steel alloys[66-72], aluminum alloys to magnesium alloys[73], and steel alloys to copper[74]. Each metal group had different combinations of optimized parameters. For each metal group, the ranking began on each combination of optimized parameters and then aggregated the weights of parameters of all combinations to obtain the overall ranking. Second, the general rank of FSW parameters was calculated by aggregating the weights of parameters of all metal groups. The final ranking was calculated regardless of the types of metals used in these joints.

 

The ranking process runs through several sections and steps. The first section is a data collection which includes two steps. The first step is to collect the DOE optimization studies of FSW parameters of all metal groups selected. The second step is to sort the literature according to the combinations of parameters considered in the optimization process for each metal group. The second section involves the AHP for FSW parameters, also including two steps (steps 3 and 4). The third step is to identify the criteria utilized to rank the parameters. The fourth step is to apply the AHP process for each metal group by establishing the relations between parameters, calculating the weight of each parameter on each combination, and ranking the parameters in each combination. The final section involves the aggregation process of weights and the overall ranking of the parameters on each metal joining group individually. Then, the general ranking of FSW parameters is obtained by aggregating the weights regardless of the metal types (aggregating the overall weights).

 

2 DATA AND METHODS

2.1 Data Collection

Literature overviews of the optimization of FSW parameters for aluminum and its alloys[2-45], magnesium alloys[47-52], steel and its alloys[53,54], copper and its alloys (i.e., brass)[55,56], dissimilar joints of aluminum to copper and their alloys[57-65], aluminum alloys to steel alloys[66-72], aluminum alloys to magnesium alloys[73], and steel alloys to copper[74] are provided in Tables 1-8. It demonstrates the selected parameters in their optimization studies, percentage of contributions % obtained, arrays used, alloys, thicknesses of materials, statistical DOE methods performed, and the efficiencies of responses (properties) acquired in these investigations. The data were gathered based on the studies that used only the design of experimental arrays to optimize these parameters on a response representing the quality of the joint. The constraints used to gather the data are summarized in Table 9. The optimum values obtained from the optimization studies for FSW parameters to maximize the responses are collected in Appendix 1.

 

Table 1. An Overview of the Literature on the Optimization for FSW Parameters of Aluminum and Its Alloys

* Discover abbreviations on the complete list of abbreviations.

 

Table 2. An Overview of the Literature on the Optimization for FSW Parameters of Magnesium Alloys

 

Table 3. An Overview of the Literature on the Optimization for FSW Parameters of Steel and Its Alloys

 

Table 4. An Overview of the Literature on the Optimization for FSW Parameters of Copper and Its Alloys (i.e., brass)

* Discover abbreviations on the complete list of abbreviations.

 

Table 5. An Overview of the Literature on the Optimization for FSW Parameters of Dissimilar Joints of Aluminum to Copper and Their Alloys

 

Table 6. An Overview of the Literature on the Optimization for FSW Parameters of Aluminum Alloys to Steel Alloys

 

Table 7. An Overview of the Literature on the Optimization for FSW Parameters of Aluminum Alloys to Magnesium Alloys

 

Table 8. An Overview of the Literature on the Optimization for FSW Parameters of Steel Alloys to Copper

 

Table 9. A Summary of Collected Data Limitations for Metal Joining Groups

UTS : Ultimate tensile strength; HRB: Hardness B.

 

The optimization studies were collected and then arranged in combinations according to the similarity of selected parameters to be optimized. This is accomplished for each metal joining group. The combinations of the considered parameters are listed in Table 10.

 

Table 10. A Detailed List of the Combinations of the Optimized Parameters for Each Metal Joining Group

* Discover abbreviations on the complete list of abbreviations.

 

2.2 AHP for FSW Parameters

The more common uses of AHP are in industry and academia. It has earned considerable attention in numerous fields, such as machine or supplier selection and other resource prioritization. At every level of the hierarchy, by comparing each parameter or alternative with the other, AHP attempts to determine the weight of each component of a finding to select, evaluate, rank, or/and make a decision[77].

 

In summary, the AHP involves seven procedures as follows:

1. Generate pairwise comparisons based on the degree of importance of parameters relative to each other (A).

2. Calculate the normalizing matrix. Each component is divided by the sum of its column. The sum of each column is 1.

3. Estimate the weight by averaging across the rows in the normalizing matrix (W).

4. Multiply each column of the pairwise comparison matrix by the corresponding weight Equation (1).

5. Calculate the eigenvector λ by Equation (2) and divide it by N (number of parameters) to obtain λAV as given in Equation (3).

6. Calculate the consistency index (CI) as given in Equation (4).

7. Check the Saaty ratio for consistency as given in Equation (5). If the ratio exceeds 0.1, the determination is considered to be significantly close to simple random and may require repeated estimation[77].

 

 

The percentages of contributions % and the degrees obtained from the ANOVA of the parameters are the two criteria used in the ranking process. Reliance on only the contribution percentages of the parameters extracted from the ANOVA tables may be inaccurate due to statistical errors obtained in these studies. Some of these studies exhibit relatively high statistical errors due to reasons such as inappropriate orthogonal arrays, neglect of the interaction between parameters, or/ and setting improper levels. Therefore, the second criterion is developed based on the degrees of the parameters in their ANOVA tables. As given in Table 11, each parameter is given a normalized contribution according to its degree.

 

Table 11. Normalizing Rate Based on the Number of Parameters

 

The second normalized contribution is combined with the actual contribution percentage % to give the general rating or the average overall contribution. The pairwise comparisons are generated according to the differences between the overall contribution of the parameters. Table 12 illustrates an example of these measurements for one combination of the parameters, e.g., RS, TS, and AF. This combination relates to aluminum and its alloys joining group. For all other combinations of the optimized parameters, the measurements were similarly calculated.

 

Table 12. The Differences in Overall Contributions between RS, TS, and AF Based on the Criteria Used

 

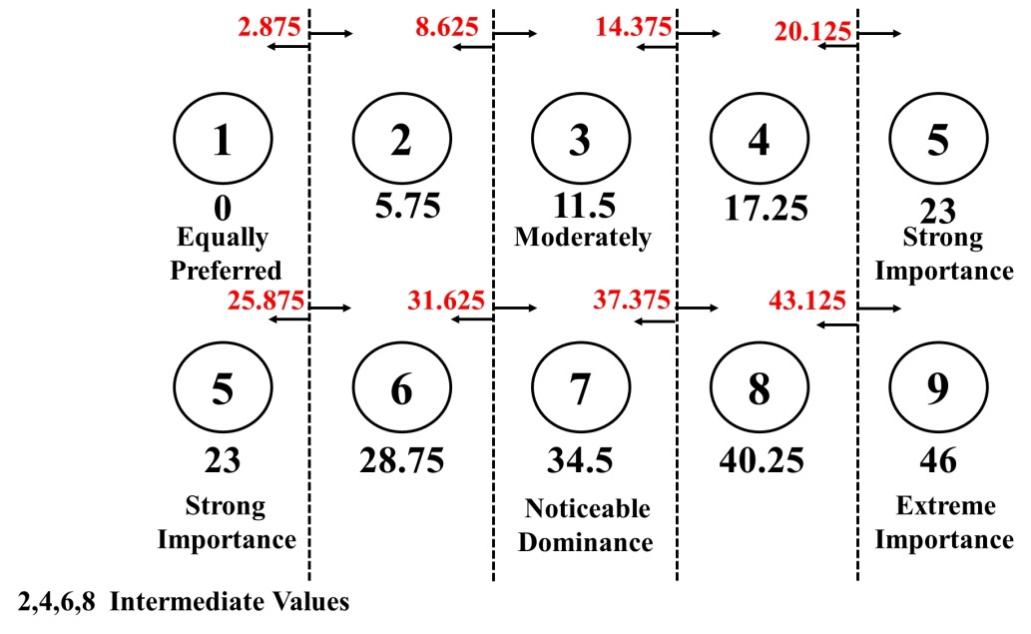
After all measurements, the differences in the overall contribution percentages % between parameters range from 0 to 46 %. The degrees based on AHP between equal importance (1) and absolutely more important (9) are built according to these differences in the overall contributions. According to the measurements, the contribution of 5.75 % is the transition value from a degree to a higher one of importance. If the value of the difference lies between two grades, the selection of the appropriate degree is determined based on the convergence of the contribution value from this degree. Figure 1 shows the AHP degrees of importance and their corresponding contributions. As depicted in Figure 1, between degrees 1 and 2 as an example, if the difference in the overall contribution is more than 2.875%, the proper degree of the relation between two parameters is 2, and vice versa.

 

Figure 1. The AHP degrees of importance and their corresponding percentages of contributions.

 

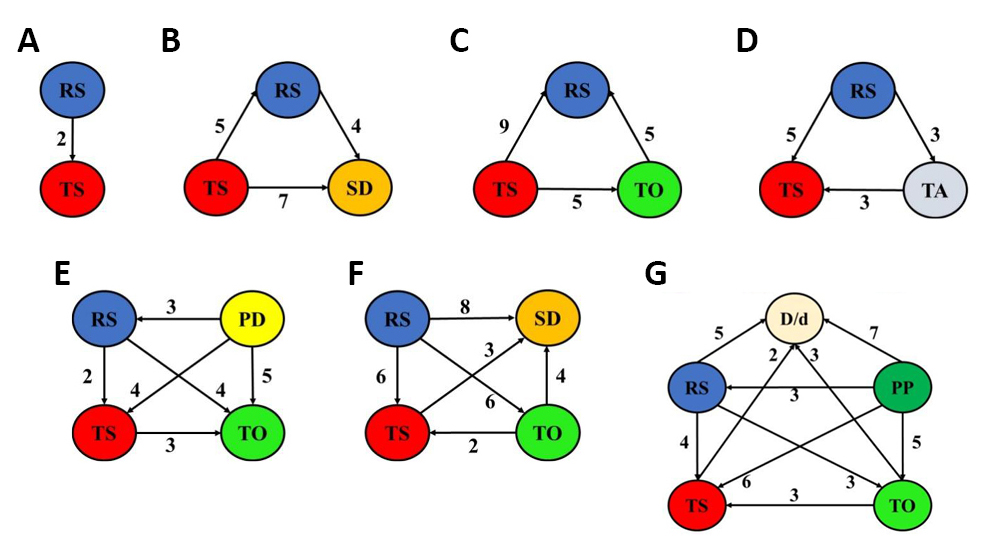
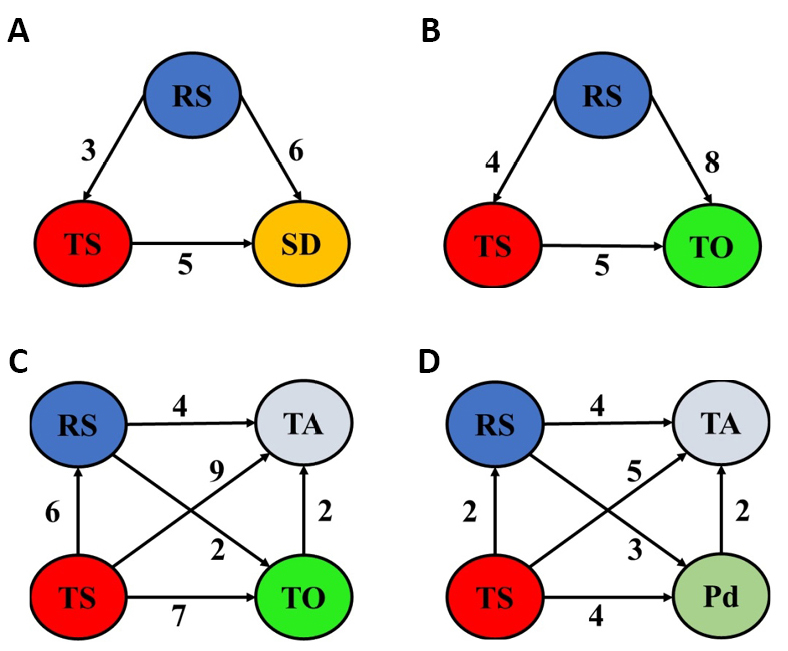
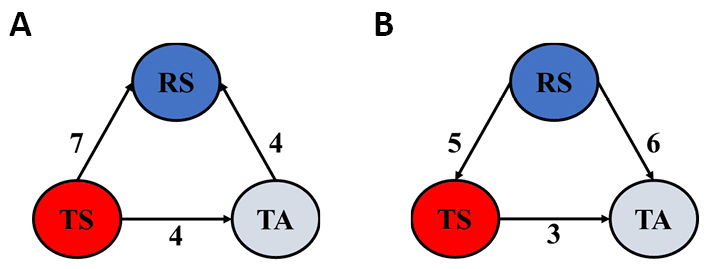
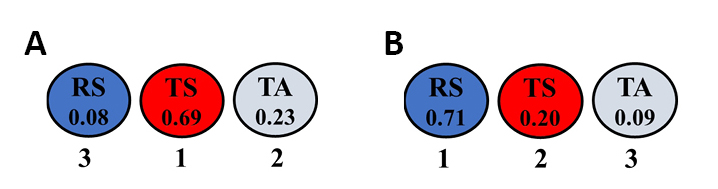
As represented in Table 12, the difference in overall contribution between RS and TS is 12.8725%, so the grade of importance between RS and TS is 3, indicating that the RS somewhat more important than TS. The AHP pair comparisons and normalized matrix for the combination of RS, TS, and AF are given in Tables 13 and 14. The other relationships are expressed in the same manner. The weights of RS, TS, and AF are 0.589, 0.159, and 0.251, respectively. In this combination, the ranks of RS, TS, and AF are 1, 3, and 2, respectively. The consistency ratios were checked using the division between consistency index and random index (given in Table 15). The consistency ratio CR scores 0.027 (<0.1) suggesting a consistent comparison. In the following Figures, the relation (degree of importance) between two parameters is depicted by an arrow or a line. The arrow goes from the parameter with a higher importance degree over another. Also, the line denotes an equal degree of importance between the parameters. The relations and the degrees of importance of the optimized two-three, four, five, and seven parameters for aluminum and its alloys are depicted in Figures 2-5. The relations and the degrees of importance of the optimized three, four, and five parameters for magnesium alloys are in Figure 6. Also, the relations and the degrees of importance of the optimized eight parameters are in Figure 7. The relations and the degrees of importance of the optimized three parameters for steel and its alloys are depicted in Figure 8A. The relations and the degrees of importance of the optimized three parameters for copper and its alloys (i.e., brass) are in Figure 8B. The relations and the degrees of importance of the optimized two, three, four, and five parameters for dissimilar joints of aluminum to copper and their alloys are depicted in Figure 9. The relations and the degrees of importance of the optimized two, three, four, and five parameters for aluminum alloys to steel alloys are depicted in Figure 10. The relations and the degrees of importance of the optimized three parameters for aluminum alloys to magnesium alloys are in Figure 11A. The relations and the degrees of importance of the optimized three parameters for steel alloys to copper are in Figure 11B.

 

Table 13. AHP Pairs Comparison in the Combination of RS/TS/AF from Aluminum and Its Alloys Group

 

Table 14. Normalized Matrix for RS/TS/AF Combination from Aluminum and Its Alloys Group

 

Table 15. Random Index (RI) Table

 

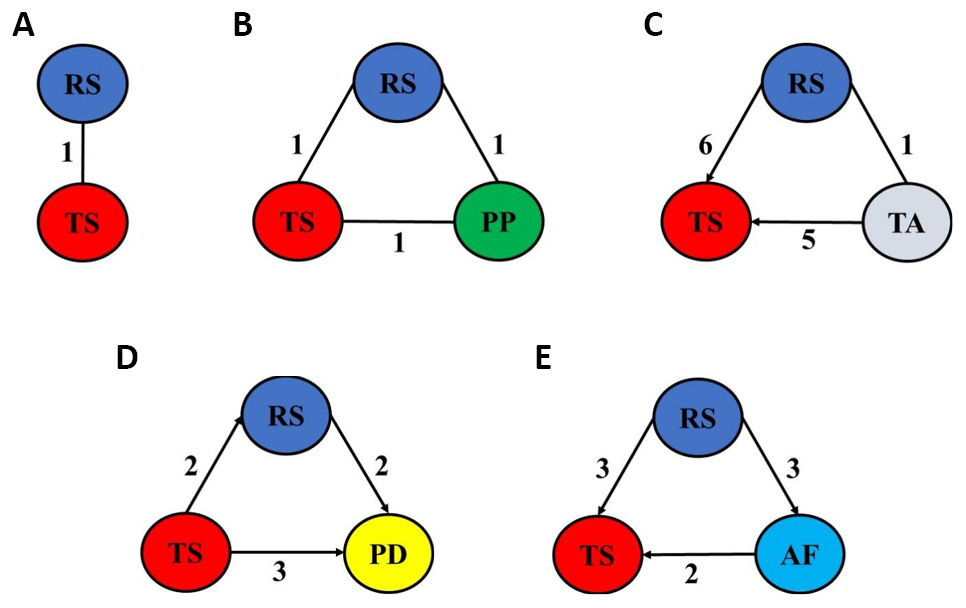
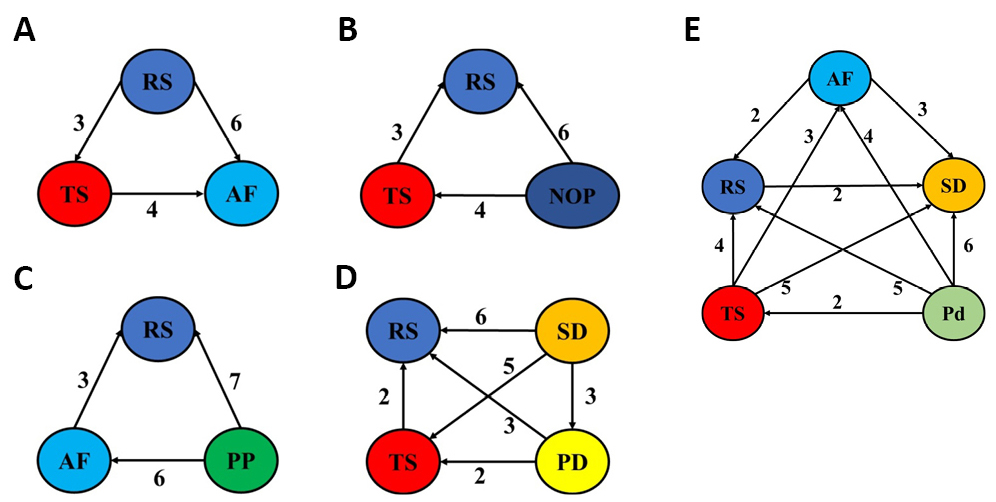
Figure 2. The relations and the degrees of importance of the optimized two-three process parameters for aluminum and its alloys: RS/TS (A), RS/TS/PP (B), RS/TS/TA (C), RS/TS/PD (D), and RS/TS/AF (E).

 

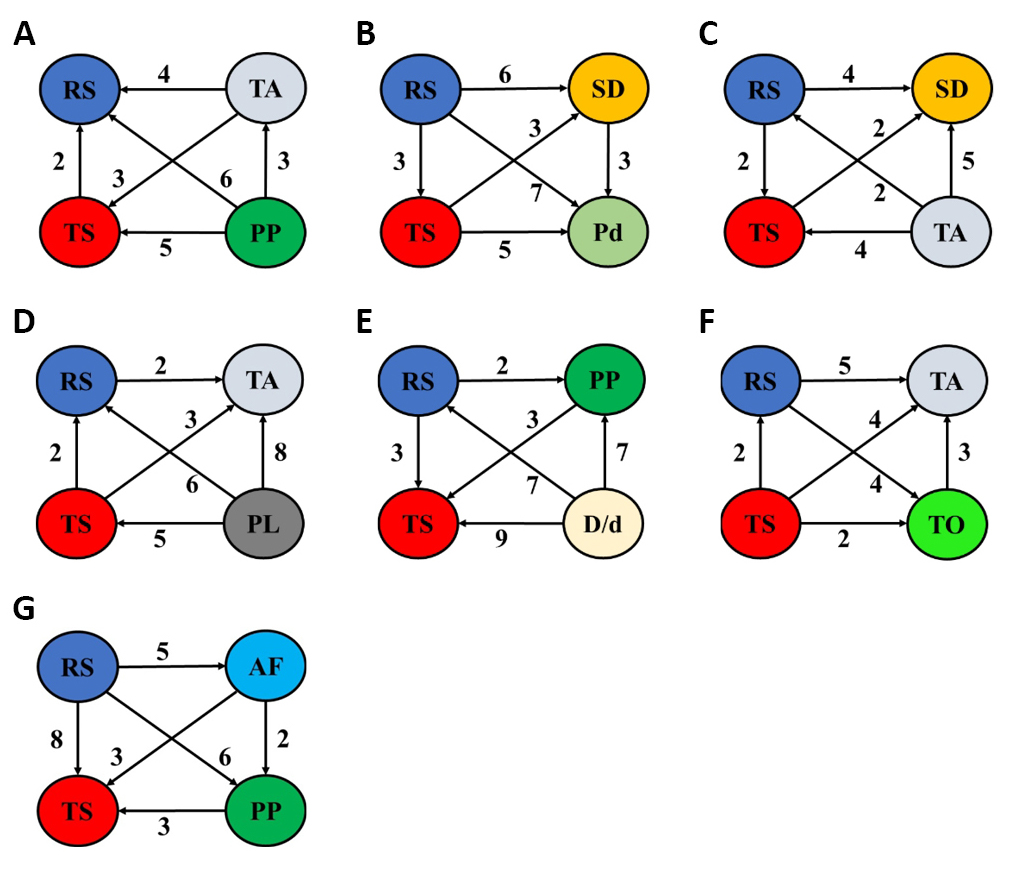
Figure 3. The relations and the degrees of importance of the optimized four process parameters for aluminum and its alloys: RS/TS/PP/TA (A), RS/TS/SD/Pd (B), RS/TS/TA/SD (C), RS/TS/TS/PL (D), RS/TS/PP//D/d ratio (E), RS/TS/TA/TO (F), and RS/TS/AF/PP (G).

 

Figure 4. The relations and the degrees of importance of the optimized five process parameters for aluminum and its alloys: RS/TS/PP/TA/TO (A), RS/TS/TA/PL//D/d ratio (B), and RS/TS/AF/PP/TA (C).

 

Figure 5. The relations and the degrees of importance of the optimized seven process parameters for aluminum and its alloys: RS/TS/PP/TA/PD/BMS//D/d ratio.

 

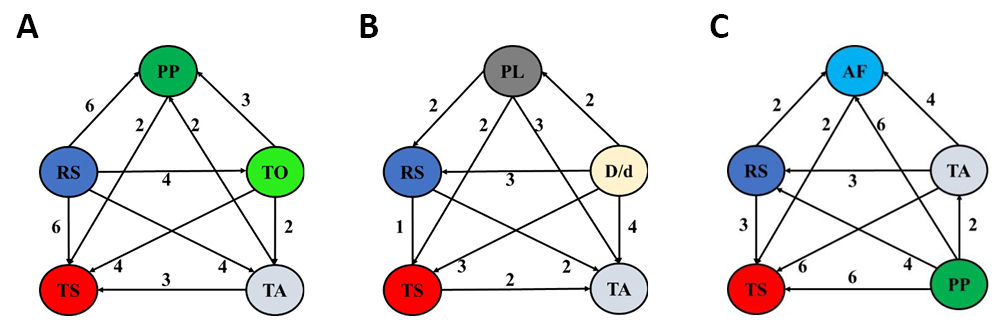
Figure 6. The relations and the degrees of importance of the optimized three-four-five process parameters for magnesium and its alloys: RS/TS/AF (A), RS/TS/NOP (B), RS/TS/PP(C), RS/TS/SD/PD (D), and RS/TS/AF/SD/Pd (E).

 

Figure 7. The relations and the degrees of importance of the optimized eight process parameters for magnesium and its alloys: RS/TS/PP/TA/SD/Pd/SC/PD.

 

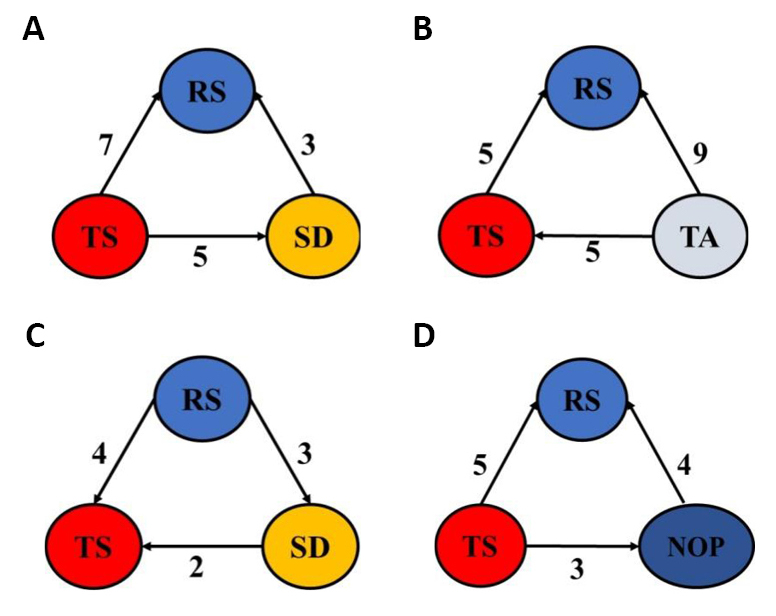
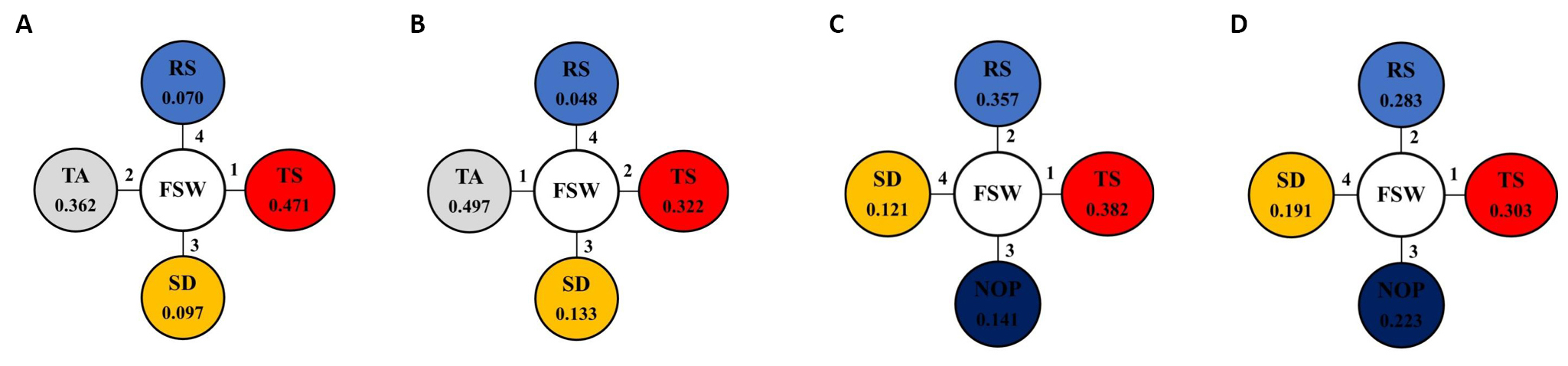
Figure 8. The relations and the degrees of importance of the optimized three process parameters for steel and its alloys: RS/TS/SD (A), and RS/TS/TA (B), and for copper and its alloys: RS/TS/SD (C), and RS/TS/NOP (D).

 

Figure 9. The relations and the degrees of importance of the optimized two-three-four-five process parameters for dissimilar aluminum-to-copper and their alloys: RS/TS (A), RS/TS/SD (B), RS/TS/TO (C), RS/TS/TA (D), RS/TS/PD/TO (E), RS/TS/SD/TO (F), and RS/TS/PP//D/d ratio/TO (G).

 

Figure 10. The relations and the degrees of importance of the optimized two-three-four-five process parameters for dissimilar aluminum-to-steel and their alloys: RS/TS/SD (A), RS/TS/TO (B), RS/TS/TA/TO (C) and RS/TS/TA/Pd (D).

 

Figure 11. The relations and the degrees of importance of the optimized three process parameters for dissimilar aluminum-to-magnesium and their alloys: RS/TS/TA (A) and for dissimilar steel-to-copper and their alloys: RS/TS/TA (B)

 

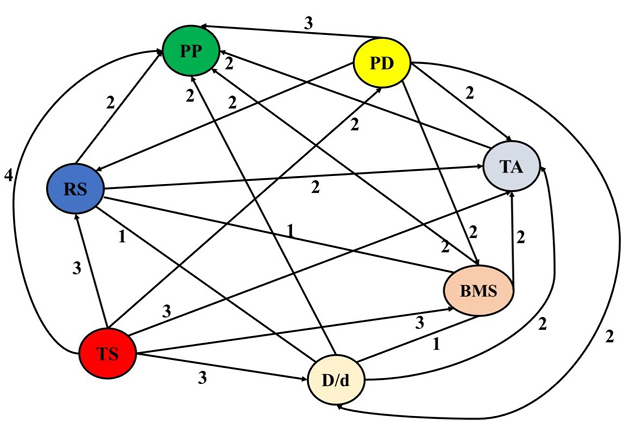
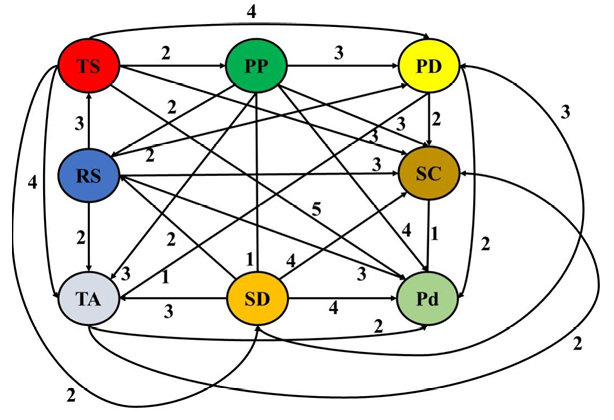
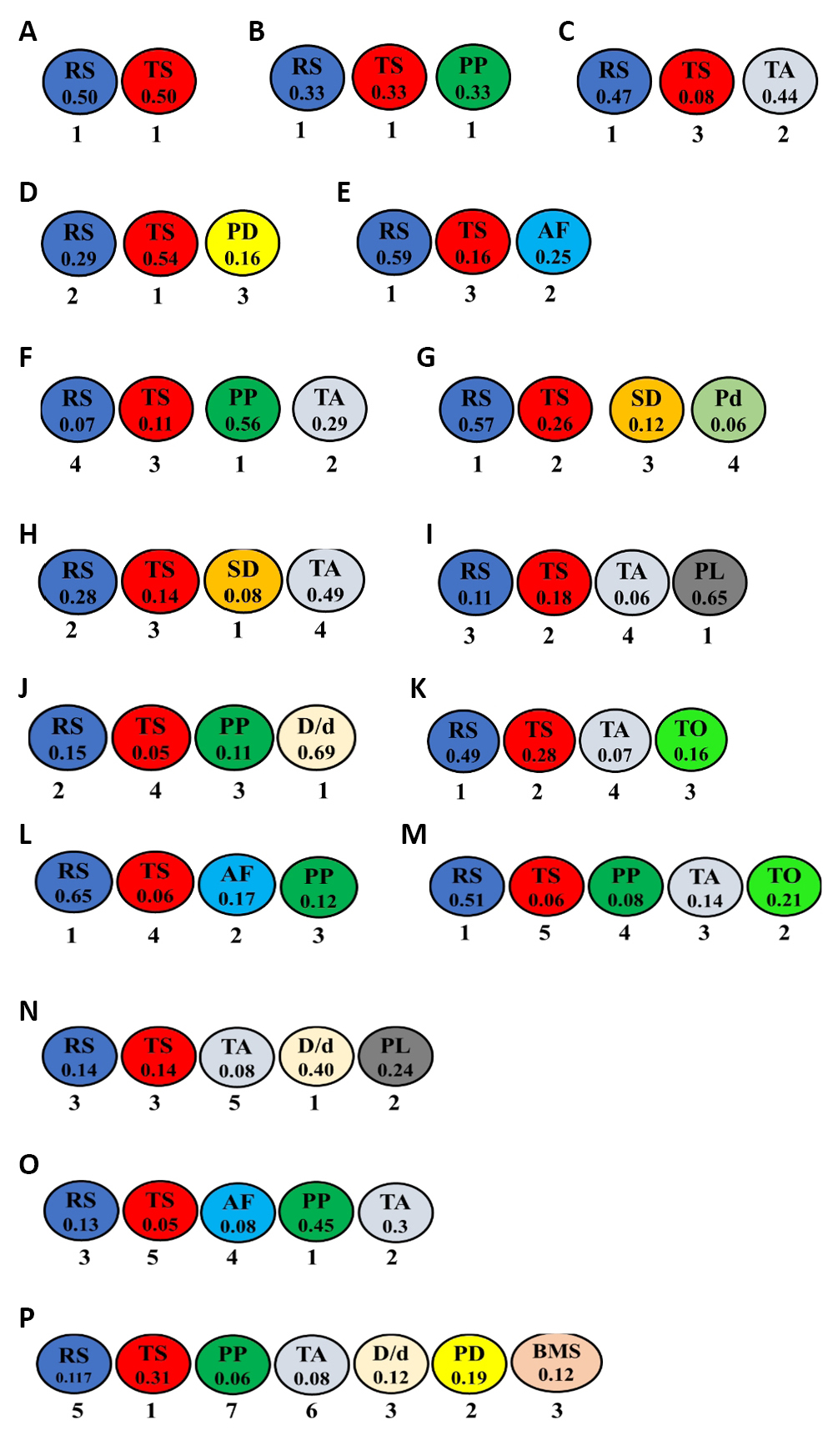
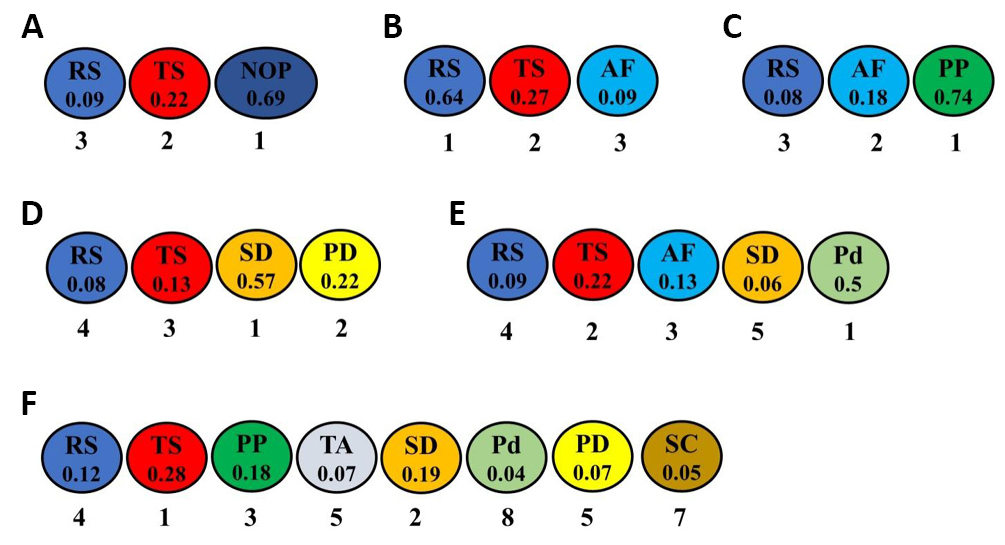
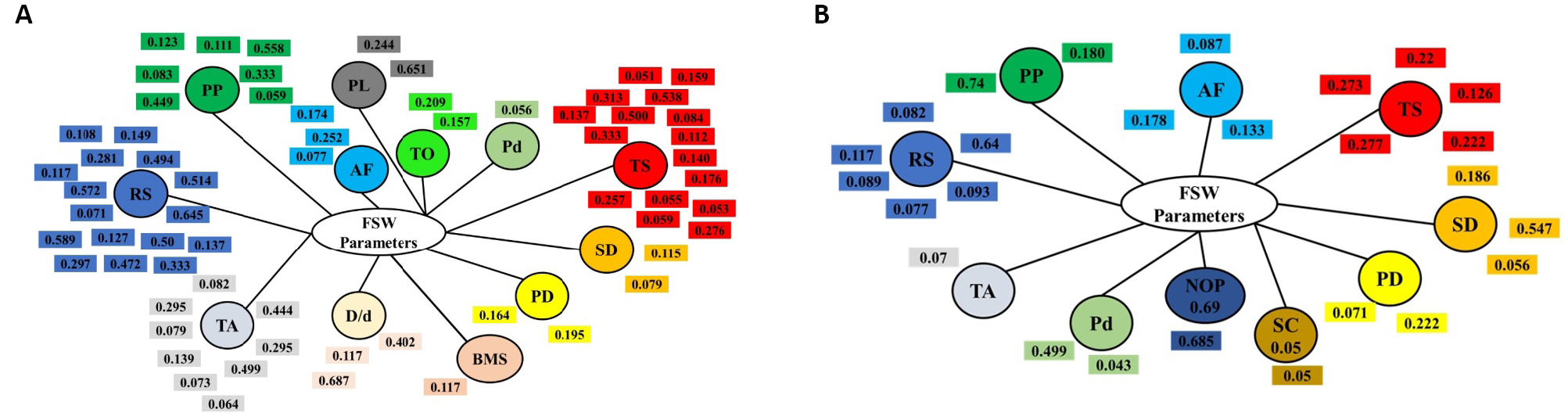
The ranking and estimated weights of all optimized parameters in all sixteen combinations for aluminum and its alloys are given in Figure 12. The ranking and estimated weights of all optimized parameters in all six combinations for magnesium alloys are in Figure 13. The ranking and estimated weights of all optimized parameters in the two combinations for steel and its alloys are demonstrated in Figure 14A and 14B. The ranking and estimated weights of all optimized parameters in the two combinations for copper and its alloys (i.e., brass) are shown in Figure 14C and 14D. The ranking and estimated weights of all optimized parameters in all seven combinations for dissimilar joints of aluminum to copper and their alloys are shown in Figure 15. The ranking and estimated weights of all optimized parameters in all four combinations for aluminum alloys to steel alloys are given in Figure 16. The ranking and estimated weights of optimized parameters in the available combination for aluminum alloys to magnesium alloys are demonstrated in Figure 17A. The ranking and estimated weights of optimized parameters in the available combination for steel alloys to copper are demonstrated in Figure 17B. A comparison between the ranks of the parameters in the optimized combinations for all metal groups is illustrated in Table 14.

 

Figure 12. The ranks and estimated weights of all optimized parameters in the sixteen combinations for aluminum and its alloys: RS/TS (A), RS/TS/PP (B), RS/TS/TA (C), RS/TS/PD (D), RS/TS/AF (E), RS/TS/PP/TA (F), RS/TS/SD/Pd (G), RS/TS/TA/SD (H), RS/TS/TS/PL (I), RS/TS/PP//D/d ratio (J), RS/TS/TA/TO (K), RS/TS/AF/PP (L), RS/TS/PP/TA/TO (M), RS/TS/TA/PL//D/d ratio (N), RS/TS/AF/PP/TA (O), and RS/TS/PP/TA/PD/BMS//D/d ratio (P).

 

Figure 13. The ranks and estimated weights of all optimized parameters in the six combinations for magnesium and its alloys: RS/TS/NOP (A), RS/TS/AF (B), RS/TS/PP (C), RS/TS/SD/PD (D), RS/TS/AF/SD/Pd (E), and RS/TS/PP/TA/SD/Pd/SC/ PD (F).

 

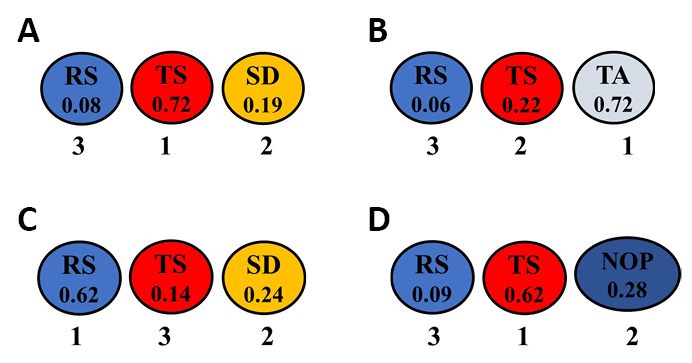
Figure 14. The ranks and estimated weights of all optimized parameters in the two combinations for steel and its alloys: RS/TS/SD (A), and RS/TS/TA (B) and for copper and its alloys: RS/TS/SD (C), and RS/TS/NOP (D).

 

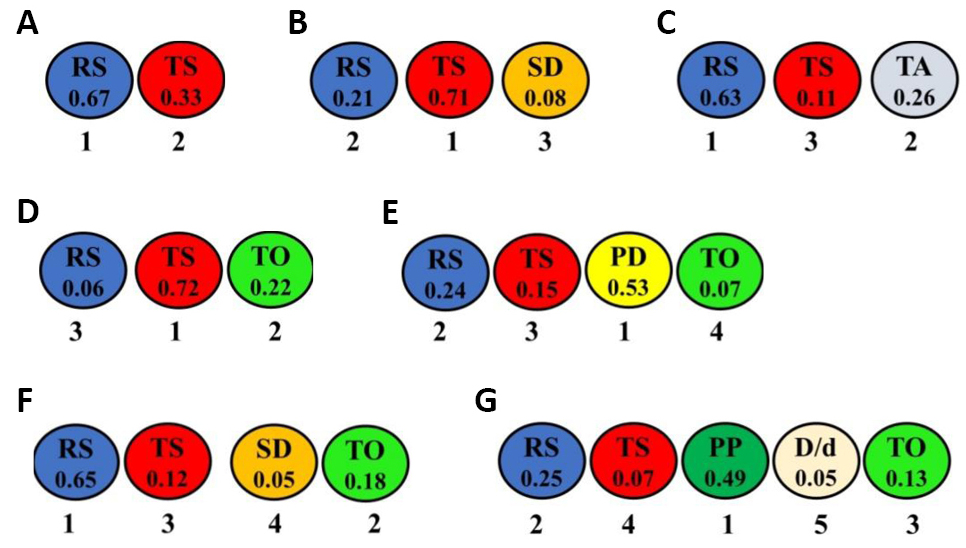
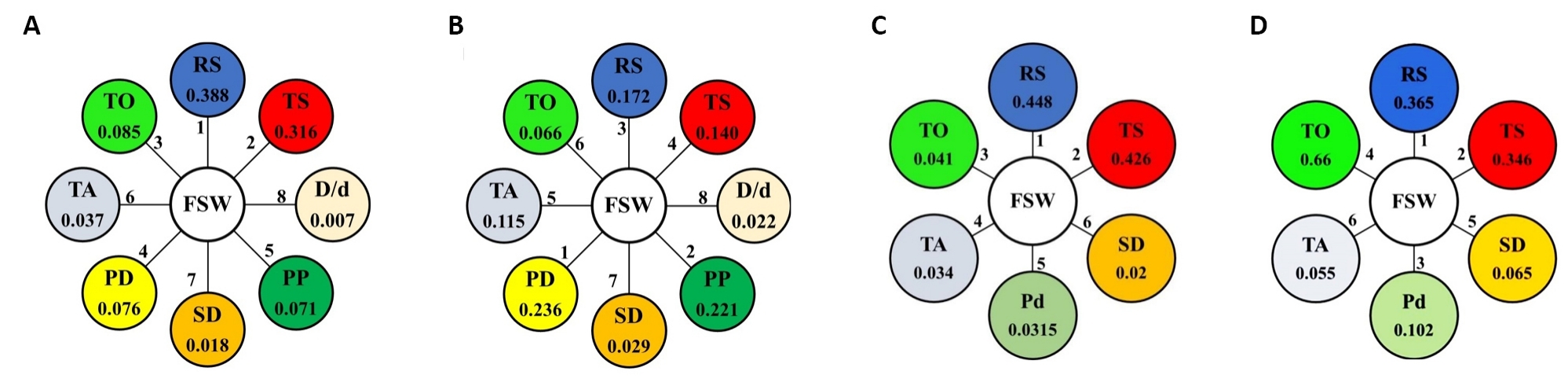
Figure 15. The ranks and estimated weights of all optimized parameters in the seven combinations for dissimilar aluminum-to-copper and their alloys: RS/TS (A), RS/TS/SD (B), RS/TS/TA (C), RS/TS/TO (D), RS/TS/PD/TO (E), RS/TS/SD/TO (F), and RS/TS/PP//D/d ratio/TO (G).

 

Figure 16. The ranks and estimated weights of all optimized parameters in the four combinations for dissimilar aluminum-to-steel and their alloys: RS/TS/TO (A), RS/TS/SD(B), RS/TS/TA/Pd (C), and RS/TS/TA/TO (D).

 

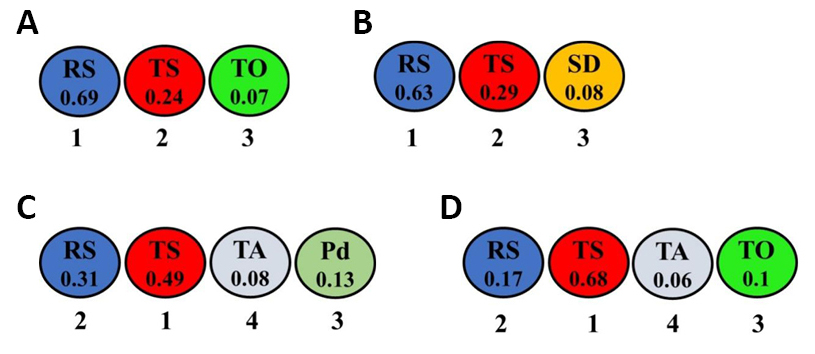
Figure 17. The ranks and estimated weights of optimized parameters for dissimilar aluminum-to-magnesium and their alloys: RS/TS/TA (A) and for dissimilar steel-to-copper and their alloys: RS/TS/TA (B).

 

Table 16. A Comparison between the Ranks of the Parameters in the Optimized Combinations for All Metal Groups

 

2.3 Weights Aggregation of FSW Parameters

Herein, the goal is to aggregate the weights of each parameter from all relations and combinations for each metal group separately. Figures 18A-19B illustrate the aggregation process of weights of the twelve parameters optimized for aluminum and its alloys, the ten parameters optimized for magnesium and its alloys, the four parameters optimized for steel and its alloys, and the four parameters optimized for copper and its alloys, respectively. For the dissimilar joining of metals, Figure 20A and 20B illustrates the aggregation process of weights of the eight parameters optimized for dissimilar aluminum-to-copper and their alloys and the six parameters optimized for dissimilar aluminum-to-steel and their alloys, respectively.

 

Figure 18. The aggregation of weights of the twelve FSW parameters optimized for aluminum and its alloys (A) and the aggregation of weights of the ten FSW parameters optimized for magnesium and its alloys (B).

 

Figure 19. The aggregation of weights of the four FSW parameters optimized for steel and its alloys (A) and for copper and its alloys (B).

 

Figure 20. The aggregation of weights of the eight FSW parameters optimized for dissimilar aluminum-to-copper and their alloys (A) and the aggregation of weights of the six FSW parameters optimized for dissimilar aluminum-to-steel and their alloys (B).

 

3 RESULTS

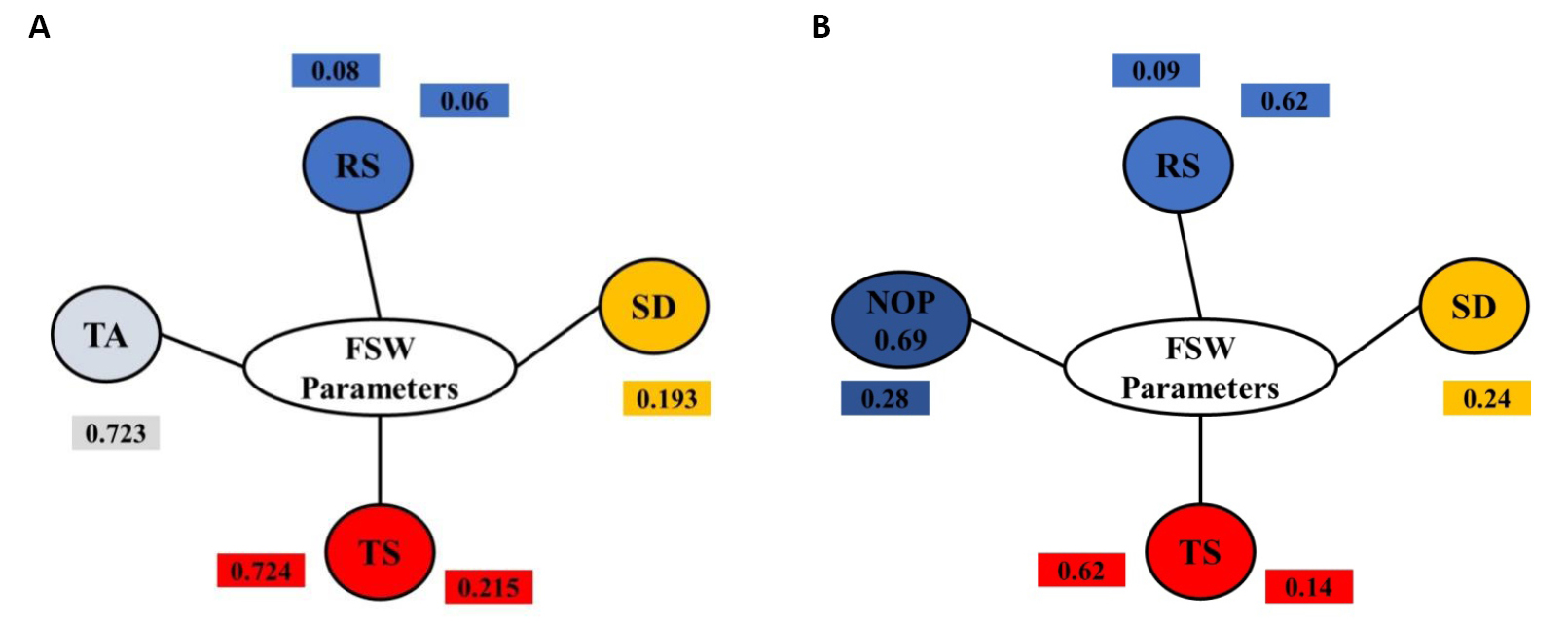
3.1 The Final Ranking of FSW Parameters

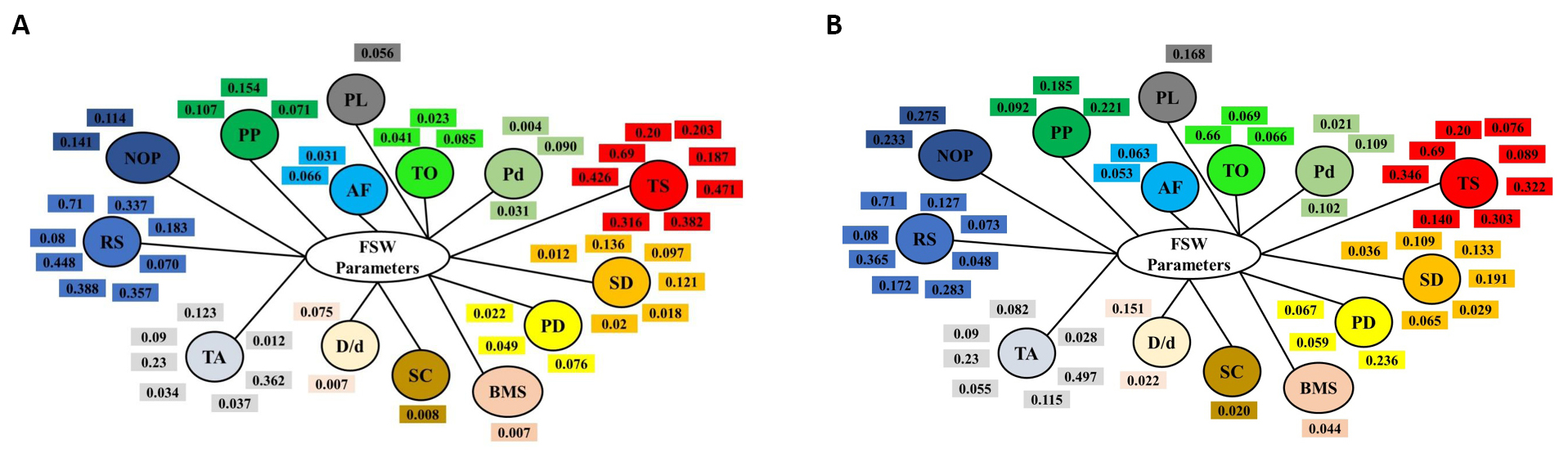
After collecting all weights of the parameters, there was a deficiency in the number of collected weights of all parameters except for RS and TS, which were optimized in all combinations and rankings. Thus, to increase the accuracy, the overall ranking was estimated in following two manners. First, the ranking of a parameter in its metal group is based on its total weight divided by the total number of combinations in this metal group, called weight criterion 1. Second, the ranking of a parameter is based on its total weight divided by the number of combinations containing this parameter only in its metal group, called weight criterion 2. The two criteria were used to rank the parameters in each metal group. The overall ranking and weights of the parameters optimized for aluminum and its alloys, magnesium and its alloys, steel and its alloys, copper and its alloys, dissimilar aluminum-to-copper and their alloys, and dissimilar aluminum-to-steel and their alloys are shown in Figures 21-23. The overall ranking and weights of the parameters in the metal groups of dissimilar aluminum-to-magnesium and dissimilar steel-to-copper are identical to the estimated in its combination (Figure 17). This is attributed to the use of a single combination of parameters in these groups. Despite the alterations in the order of the parameters based on the type of metal, the RS, TS, TA, and PP are considered the most dominant parameters to maximize the responses of many various metal joints. It is also insufficient to control these parameters only. Some other parameters besides the previously mentioned should be handled according to their ranking for each metal type. It should control the D/d ratio and PL for the joints of aluminum and its alloys. The number of passes and SD should be handled for the joints of magnesium alloys. TO is significant in both aluminum-to-steel joints and aluminum-to-copper joints. A comparison of the overall ranking of the parameters for all metal groups is given in Table 17.

 

Figure 21. The final ranks and weights of the parameters optimized for aluminum and its alloys according to weight criterion 1 (A) and weight criterion 2 (B), and for magnesium and its alloys according to weight criterion 1 (C) and weight criterion 2 (D).

 

Figure 22. The final ranks and weights of the parameters optimized for steel and its alloys according to weight criterion 1 (A) and weight criterion 2 (B), and the final ranks and weights of the parameters optimized for copper and its alloys according to weight criterion 1 (C) and weight criterion 2 (D).

 

Figure 23. The final ranks and weights of the parameters optimized for dissimilar aluminum-to-copper and their alloys according to weight criterion 1 (A) and weight criterion (B) and the final ranks and weights of the parameters optimized for dissimilar aluminum-to-steel and their alloys according to weight criterion 1 (C) and weight criterion 2 (D).

 

Table 17. A Comparison of the Overall Ranking of the Parameters for All Metal Groups

(1st No./2nd No.)*: 1st: first rank using weight criterion 1, 2nd: second rank using weight criterion 2

 

It is required to maintain these parameters at optimal levels depending on the process and production conditions, which requires pre-testing the levels of the parameters on the desired process. Based on the literature, the optimal levels (L) of the parameters were collected and formulated on a domain for each type of metal joint. The domain involves two limits, namely upper level, and lower levels. Operating out of the limits will drastically affect the responses (properties). The domains of the optimal levels are expressed in Equations (6)-(13) for aluminum, magnesium, steel, copper/brass, aluminum to copper, aluminum to steel, aluminum to magnesium, and steel to copper. The domain involving a limit should remain constant when testing levels.

 

 

Pin profile: S-Square, TC-Threaded Cylinder, H-Hexagonal

Pin length*: related to the thickness of the metal used, normally less than the metal thickness about 0.2-0.3 mm.

Units: RS: RPM; TS: min/min; AF: KN; PP: Shape; TA: º; D/d ratio: - ; SD: mm; Pd: mm; PD: mm; PL: mm; TO: mm; BMS: CW, CCW; NOP: #; SC: º.

 

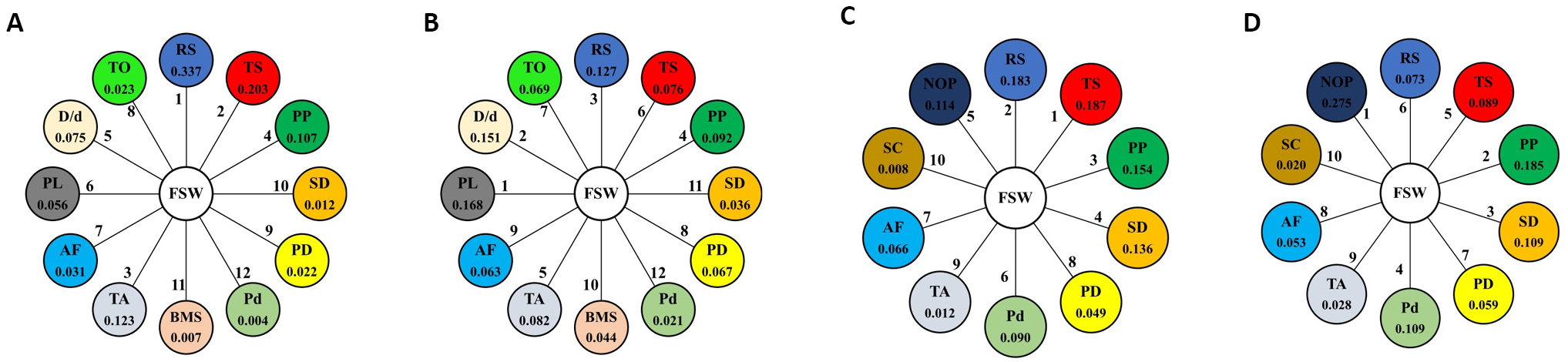
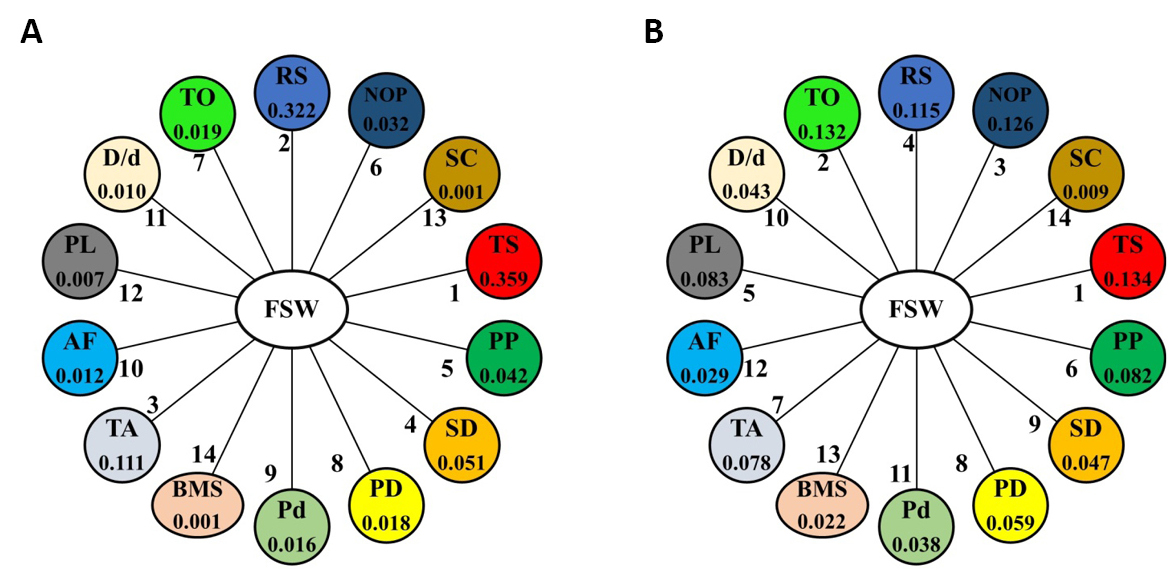
3.2 The General Ranking of FSW Parameters

The final step was to develop the general ranking of the FSW parameters regardless of the type of metal used. This was done by aggregating the weights of each parameter from all metal groups and generating the general ranking. Figure 24A and 24B illustrates the aggregation process of weights of all FSW parameters optimized using weight criteria 1 and 2. The general ranking and final weights of the FSW parameters are shown in Figure 25. Despite the changes in the order of the parameters based on the different criteria used to estimate the weights, the parameters could be classified into three categories, namely highly significant, moderately significant, and insignificant. TS, RS, TA, and PP were highly significant parameters that require careful control to maximize the responses. The total contributions of these parameters to the output were 83.4% and 40.9% based on the criteria used to estimate weights. The insignificant parameters were the D/d ratio, BMS, SC, AF, and Pd, with a total contribution to the response between 4% and 14%. In between, there are moderately significant parameters. These parameters are the number of passes, TO, SD, PD, and PL. The failure to achieve the target specified in the standard by controlling only highly significant parameters signifies a need for further modification of the FSW process. Thus, parameters of moderate significance are potential parameters that need to be controlled to achieve the target. To limit the selection between these parameters, reference should be made to the type of metal used and then the parameters with the greatest impact on the metal are selected, i.e. controlling PL for aluminum, NOP, and SD for magnesium, and TO for aluminum-to-steel and aluminum-to-copper.

 

Figure 24. The aggregation of weights of the fourteen FSW parameters optimized for the general ranking using weight criterion 1 (A) and weight criterion 2 (B).

 

Figure 25. The general ranking and final weights of the FSW parameters optimized according to (A) weight criterion 1 and (B) weight criterion 2.

 

4 DISCUSSION AND CONCLUSION

The data gathered from literature to be used in the ranking process were the contribution percentages and the degrees of the parameters obtained from the ANOVA of experimental studies. The data were sorted as per the type of metals used in the FSW joints. The weights of parameters were computed for each metal group individually and then aggregated to achieve the general ranking.

 

The results can be summarized as follows:

1) The parameters were classified into three categories, namely highly significant, moderately significant, and insignificant.

2) TS, RS, TA, and PP are highly significant parameters that necessitate careful control to maximize the responses.

3) The total contributions of the highly significant parameters to the output were 83.4% and 40.9% based on the two criteria used to estimate weights.

4) The insignificant parameters were the D/d ratio, BMS, SC, AF, and Pd.

5) The insignificant parameters may be ignored due to a low contribution of 14% or 4%, obtained from the two manners used for weight estimation.

6) The moderately significant parameters were the number of passes, TO, SD, PD, and PL.

7) The failure to achieve the target specified in the standard by controlling only highly significant parameters signifies a need for further modification of the FSW process. Thus, parameters of moderate significance are potential parameters that need to be controlled to achieve the target.

8) To limit the selection between these parameters, reference should be made to the type of metal used and then the parameters with the greatest impact on the metal are selected, i.e. controlling PL for aluminum, NOP, and SD for magnesium, and TO for aluminum-to-steel and aluminum-to-copper.

9) The optimal levels of the parameters were formulated on equations having a domain for each type of metal. Operating out of the limits will drastically affect the responses (properties).

 

Acknowledgements

Not applicable.

 

Conflicts of Interest

The authors declared no conflict of interest.

 

Author Contribution

Mohamed MAE was responsible for conceptualization, data curation, project administration, formal analysis, investigation, methodology, software, figures creation, writing-original draft, writing-review & editing. Abdelrahman A was responsible for software, editing, and figures creation.

 

Abbreviation List

AA, Aluminum alloy

AF, Axial Force

AHP, Analytic hierarchy process

ANOVA, Analysis of variance

AS, Advancing side

BBD, Box-Behnken design

BMS, Base metal side

CCD, Central composite design

CI, Consistency Index

CR, Consistency ratio

D/d ratio, Ratio between shoulder diameter to pin diameter

DOE, Design of experiments

FDD, Full factorial design

FSW, Friction stir welding

GRA, Gray relation analysis

LP, Number of levels and parameters

NOP, No of passes

Pd, Pin diameter

PD, Plunge depth

PL, Pin length

PP, Pin profile

RI, Random Index

RS, Rotational speed/ rpm

SC, Shoulder concavity

SC, Shoulder cavity

SD, Shoulder diameter

TA, Tilt angle

TO, Tool offset

TS, Traverse speed

UTS, Ultimate tensile strength

 

References

[14] Ghaffarpour M, Dariani BM, Kokabi AH et al. Friction stir welding parameters optimization of heterogeneous tailored welded blank sheets of aluminium alloys 6061 and 5083 using response surface methodology. P I Mech Eng B-J En, 2012; 226: 2013-2022. DOI: 10.1177/0954405412461864

 

Copyright ©2022 The Author(s). This open-access article is licensed under a Creative Commons Attribution 4.0 International License (https://creativecommons.org/licenses/by/4.0), which permits unrestricted use, sharing, adaptation, distribution, and reproduction in any medium, provided the original work is properly cited.